Abstract algebra is a field of mathematics that is used to study functions and relationships that are more general than their concrete, real-life counterparts. It is a branch of algebra that studies how numbers and other algebraic structures can be described abstractly. It is somewhat related to algebra and geometry as it deals with abstract objects like numbers and sizes.
Abstract algebra help online is vital
Did you know that Abstract Algebra is a quite later level of algebra? The subject focuses on algebraic structures. The subject is different from customary algebra, which has a quantitative-based syllabus. Abstract algebra involves studying field theory, ring theory, quotient fields, and different algebraic structure-centered material. Some key topics in abstract algebra include group theory, commutative rings, automorphisms of fields, etc. There are quite a few premium providers of abstract algebra help online.
They make a comprehensive abstract algebra tutor for scholars to help with quite a few Abstract Algebra topics. They include Algebraic Extensions, Abelian Groups, Automorphisms of Fields, Alternating Groups, Cauchy’s Theorem, Commutative Ring, Cyclic Groups, and Cyclotomic Polynomials, amongst others.
What is Abstract Algebra
Abstract Algebra is expertise relevant in numerous sorts of fields and professions in the present-day economy while solving equations. People will be astonished to hear the number of professions that necessitate a working understanding of abstract algebra to accomplish day-to-day necessities. Next, we will discuss some occupations that necessitate abstract algebra skills and some prevalent tasks.
Medical professionals must understand algebra to administer medications, distinguish pattern anomalies, fill up prescriptions, etc. The conversion of diverse drug doses is prevalent in the medicinal field. As a result, having abstract algebra solving expertise will be very useful. Mainly, prescribing diverse medicine by considering age, weight, dosage, etc., for patients is vital during crunch time.
Who is a business professional who uses abstract algebra regularly? The answer is an accountant. An accountant must be good at balancing spreadsheets, forecasting costs, and making expenses reports for his or her company. Who is one more business professional who must have a working understanding of abstract algebra? The answer is a banker. A banker must calculate taxes, interest rates, etc., for his or her clients regularly. Knowledge of abstract algebra is also vital for business proprietors. They need this to calculate revenue, run rates, the GPM, and more on behalf of their shareholders. Why do they need these calculations? The answer is to showcase development potential and protected funding and investment.
A Fitness instructor must demonstrate correct exercise procedures to his or her clients. A working understanding of abstract algebra is of help to fitness instructors to analyze the perfect exercise for their patrons. What does a Fitness instructor calculate to plan a suitable workout regime? He or she calculates an individual’s height, body fat percentage, age, weight, etc. Fitness instructors could need abstract algebra to establish the percentage of dieting and exercise to fulfill their clients’ goals.
We have all heard of the profession of Civil Engineering. Civil engineers design, scrutinize and preserve systems that the common person uses daily. Some examples of these systems are:
- Bridges
- Roads
- Tunnels
- Sewer systems
- Much more
A civil engineer must understand abstract algebra to calculate the weight that a bridge or road can endure, considering pressure from vehicles. Civil engineers have another vital thing to do. They need to predict how a bridge will endure earthquakes, wind speeds, snow, etc.
We have all heard of the profession of Architecture. An architect is a professional who makes blueprints of new buildings. Two essential qualities of an architect are accuracy and algebra. He or she needs them to get the particulars correct. Showing a height of a building, the breadth of hallways, rooms, floors, etc., requires a working knowledge of abstract algebra problems and solutions.
Next, we discuss some of the features of premium providers of abstract algebra help online.
One-on-One tutoring is a crucial feature of providers of abstract algebra help online
What does one-on-One tutoring provide students? Students get the opportunity of learning from premium tutors in a remote online classroom setting.
In the course of the One-on-One planned training sessions, the tutors aid a student with his or her coursework material. They work together on a public online classroom blackboard as they discuss the subject material over the telephone. The experienced instructors of a premium provider of abstract algebra help online deliver a learning environment featuring:
- Greatly efficient teaching
- Positive support
- Confidence developing teaching practices
What is the result? The students get success.
One-on-One tutoring – what are the advantages?
Students can avail themselves of One-on-One Tutoring in the mornings, afternoons, and evenings, all seven days a week. Thus, students get maximum flexibility.
Students do not need to take the trouble of going over to learning centers. Thus, they find this form of tutoring very convenient.
Students can easily access the One-on-One Tutoring sessions, whatever their location. All that they need is a PC and an Internet connection.
Vastly experienced and competent instructors aid students with all levels of educational coursework.
A significant advantage of One-on-One Tutoring is that the instructor-student combination is maintained for every session.
Another great advantage of One-on-One Tutoring is All-inclusive individual instruction. Thus, students can master their coursework and develop a solid general base of the concepts.
Group Tutoring
What does group tutoring provide students? Students get the chance to learn from premium instructors in a group classroom setting online. What happens during planned Group tutoring sessions?
- The teacher guides the class of scholars
- The teacher reviews coursework material on a public classroom blackboard as he or she discusses the material over a classroom conference call
Moreover, Group online classes facilitate
- Consistent exam review preparatory classes
- Student groups having common abstract algebra topics in their coursework
Group Tutoring – the Advantages
Students do not need to go down to learning centers, and this is very convenient for them
Students get the privilege of organized syllabuses throughout class sessions
Students can easily access the Group Tutoring sessions, whatever their location. All that they need is a PC and an Internet connection.
Students get to learn from incredibly experienced and competent instructors who lead the Group tutoring classes
Students can review coursework with their online classroom peers outside of planned classes
Phone Helper
An effective abstract algebra problem solver is the Phone Helper service of an online provider. Students have the chance to call knowledgeable instructors regardless of their location to get instant help with abstract algebra problems.
- This feature helps students review quite a few problems with their teacher
- This feature is very convenient for students who don’t have access to a PC. They can resort to this feature in place of One-on-One tutoring to get help
What is the foremost step to solving a Math question? A student needs to understand where he or she is getting stuck and where he or she needs assistance. A confused student can get live assignment expert abstract algebra help from a subject professional of an online service provider. He or she can get step-by-step clarifications for all questions.
A student has an easier time learning a subject like abstract algebra when he or she has a reliable advisor alongside. He or she can also solve problems and questions on an electronic whiteboard along with a tutor there to guide him or her all along. Students may require Written Help. They are free to seek support from a specialized tutor or an online provider.
Email Helper
What does Email Helper of a specialized online assignment help provider offer students of abstract algebra? Students have access to a 24-hour mode of communication. They can email, fax, and scan abstract algebra coursework problems to the specialized instructors. The instructors will complete the problems in a systematic step by step manner. They will mail back the completed problems fast so that students can learn fast.
Homework Helper
Mini One-on-One tutoring sessions are another great feature of an online assignment help provider for students of abstract algebra. What happens during a mini One-on-One tutoring session? An instructor invites a student into a remote online classroom. The instructor then aids the student with his or her abstract algebra material in the coursework. They work together visually on a public classroom blackboard even as they discuss the material on the telephone.
How is homework helper different from standard One-on-One Tutoring sessions? The sessions are shorter. Thus, students get help for a specific problem that requires instant attention.
The importance of abstract algebra notes of professional online assignment help providers
Many students of abstract algebra complain that they have a hard time following proofs in their courseware books and lectures. What would many of them do to get over this problem? While reading a theorem in a book, they would keep the book aside and explore some examples. Next, they would attempt to prove the theorem on their own. After they completed doing it or could not complete doing it, they would see the proof given in their book and match. They say that this tactic has helped them have an excellent understanding of this subject. They also say that this tactic is an excellent way of practicing for exams and assignments. Exams and assignments often ask students to prove statements that they have not witnessed before.
Several abstract algebra notes of professional online help providers help students write proofs and study proof-intensive mathematics. These notes help students who have taken or are presently taking linear algebra.
These notes have several parts. What do they discuss?
- What is proof and writing a proof?
- The central makeup of a proof
- Applying the if-then method
- Some cases where the above method isn’t directly applicable
The “group” is a central concept of abstract algebra. Groups repeat all over mathematics. The techniques of group theory have influenced several parts of algebra. Lie groups and linear algebraic groups are two subdivisions of group theory that have significantly advanced and have grown to be subject areas by themselves. Some notes offer problems that help students understand the “abstract algebra groups” concept.
Getting specialized online abstract algebra and other mathematic help online is easy
Students who think that they will benefit from specialized abstract algebra online help will be pleased to know that they can get it easily. There are quite a few premium providers that offer students flexibility and affordability. They can prove to be very helpful for students. Getting professional help online involves a few easy steps. We outline the steps below.
The first thing that students need to do is to register on the website of a provider. Signing up takes very little time.
After students have registered on a website, they must post homework requests. They are going to see a catalog of instructors that can help them.
The next step for students is reviewing offers and hiring a tutor of their choice. A student who finds a tutor may be able to click to start chatting. How does this help? The tutor will understand the student’s requirements and make a solution plan centered on the student’s timeline.
Students need to pay after they review the assignment. The websites will ask students to make a payment only after both parties have agreed.
Students will get their Homework delivered in time. Right after students make a payment, work starts. The students will be delivered the assignment within a time frame agreed upon by the students and their chosen tutor.
The corporate world today is very competitive. The importance of continuing education is more than ever for career development and occupational security. People have got to balance school and university with household and work duties. Numerous adult students are doing online degree programs. They don’t have access to teachers in a standard classroom setting. They may find learning a subject like abstract algebra more challenging. Premium providers of abstract algebra help online deploy several tutoring services. Students get effective, well-timed, and suitable teaching from the comfort of their home as and when the need arises.
Abstract Algebra
Abstract algebra begins with the group. Groups are a general and powerful structure that encompass a wide range of objects, integers, polynomials, matrices, modular arithmetic functions, geometric transformations, and much more. After spending some time learning about groups, you’re ready to move on to more specific structures and groups with extra features. Today, we’ll talk about additive groups that have a second operation, multiplication. We call these rings. Before we give the textbook definition of a ring, let’s see some examples which will motivate the idea. Consider these four sets, the integers, the real numbers, two-by-three matrices with real entries, and polynomials with complex coefficients. In arithmetic, you learn about four operations, addition, subtraction, multiplication, and division. Let’s see which operations are available for each of these sets.
Integers
For the integer Z, you can add, subtract and multiply any two integers, and you’ll get another whole number, but notice that you can’t always divide. For example, one divided by two is one half, which is not an integer. It’s a fraction. So for the integers, we only have three of the four operations from arithmetic.
Real Numbers
For the real numbers, you can add, subtract, multiply and divide any two real numbers with the standard caveat that you cannot divide by zero. So the real numbers come equipped with all four operations.
Two by Three Matrices
You can add and subtract any two such matrices for the two-by-three matrices, but you cannot multiply or divide. This is because you can only multiply two matrices when the number of columns in the first matrix is the same as the number of rows in the second matrix. For example, you can multiply a three-by-five matrix by a five by two matrix because the width of the first is equal to the height of the second. And to divide one matrix A by another B, we have to multiply A by the inverse of B, and not every matrix is convertible. So this set only has two of the four operations.
Polynomials
Finally, you can add, subtract and multiply any two polynomials, and you’ll get another polynomial. But if you divide one by another, you usually get a rational function, not a polynomial. The elements in the set of polynomials only have three of the four operations.
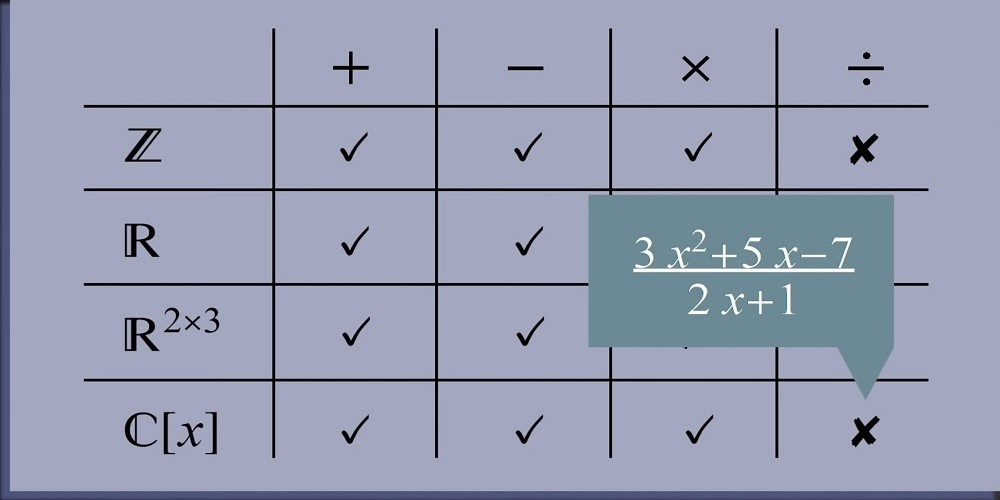
These examples show that you’ll have different operations from arithmetic available to you, depending on the set. And while in arithmetic, you learn that there are four operations in abstract algebra, we think about things a little differently. Here, there are only two operations: addition and multiplication. Let’s see why.
Examples
Two minus seven is the same as two plus negative seven, so subtraction is the same as adding a negative integer. Also, three minus negative five is the same as three plus five. So in this example, subtracting negative five is the same as adding the opposite, which is five. These examples show that subtraction is the same as adding an opposite element. But we use the word inverse instead of the opposite. So in abstract algebra, subtraction is the same as addition with inverses.
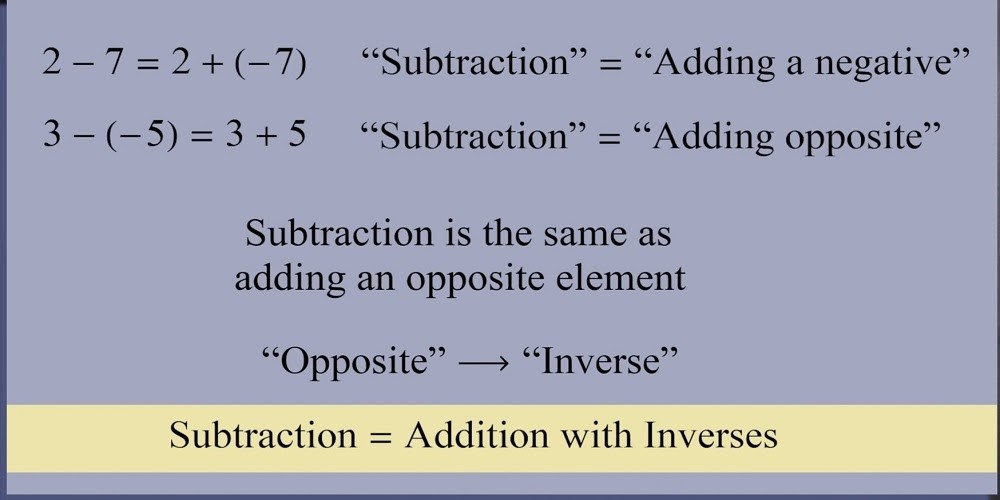
What’s the benefit of this change in thinking? Why not continue to talk about addition and subtraction? One reason is that in abstract algebra, the elements may not be numbers.
For example, the symmetric group on Three Elements is a group with six elements where each element is a permutation. What does it mean to subtract the permutation, Two, three, one? What is the negative of two, three, one? It makes more sense to talk about combining one element with the inverse of another.
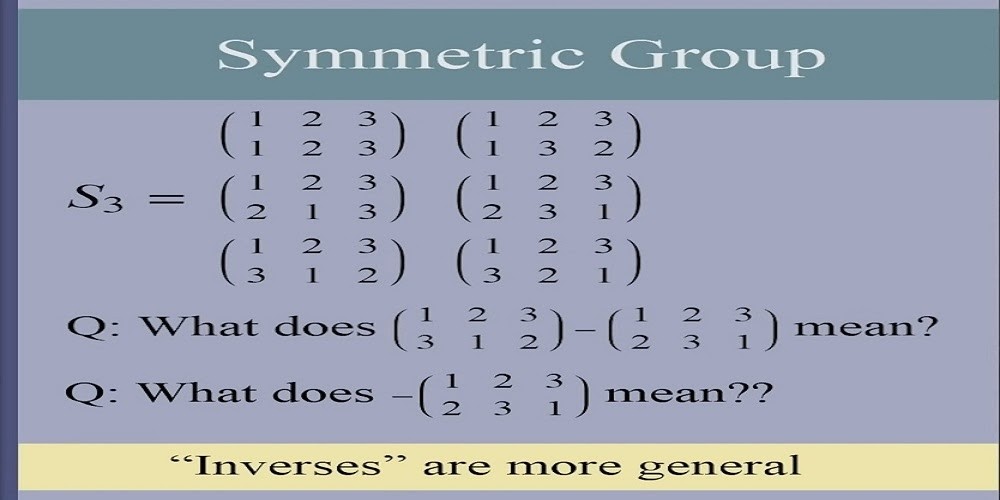
Similarly, division is multiplication with inverses. For example, three divided by five is the same as three times one fifth, and two-thirds divided by four, sevens can be written as two thirds, times seven fourths. We see for multiplication; inverse means reciprocal. Well, for addition, inverse implies the opposite. To keep things clear, we’ll often use the phrases additive inverses and multiplicative inverses.
We already have a word for a set where you can add any two elements, every element has an additive inverse, and you have the required rules from arithmetic. We call this a group. So in our four sets, all four are groups under addition. Better still, they are all commutative groups, but three of them also have multiplication. One of them has multiplication with multiplicative inverses. We need names to describe sets with these additional features when a commutative group under addition also has multiplication. We call it a ring.
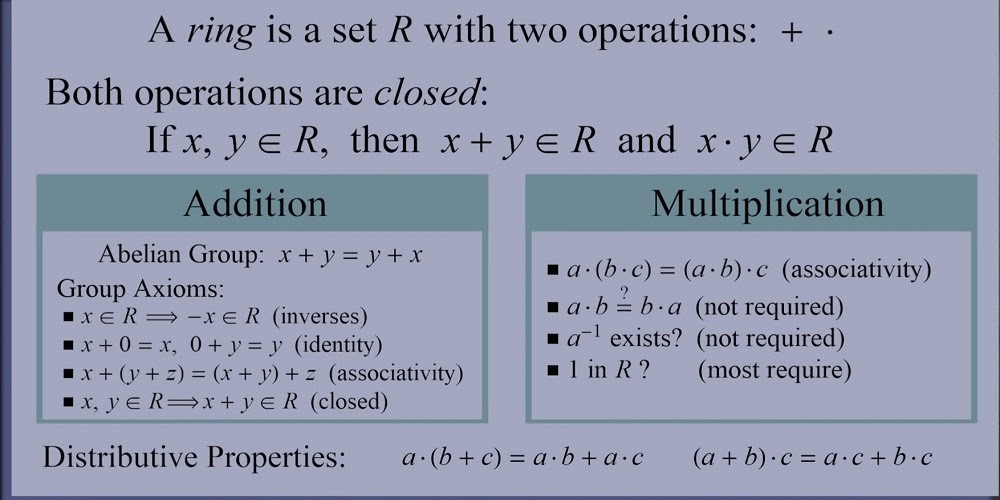
Ring
A ring is a set of elements R with two operations, addition, and multiplication. For both operations, the set is closed. This means if you add any two elements in R, you get another element in R. Similarly, if you multiply any two elements in R, you get the third element in R. In addition, the elements form an Abelian Group for multiplication, there are fewer requirements. In addition, multiplication has the associative property, but that’s it. We don’t require multiplication to be commutative. We also don’t need elements to have multiplicative inverses. There’s still some debate on whether or not a ring should have an identity element, one for multiplication. Because of this, you’ll often hear people talk about a ring with identity. This means the ring has an element one. And finally, these two operations are linked by the distributive properties. This is the official definition of a ring.
Types of Rings
There are additional names for rings depending on how close it comes to being a group under multiplication. For instance, while all rings are Abelian under addition, they are not required to be commutative under multiplication. The two by two matrices with real entries are a great example of a ring that’s not commutative. But if a ring is commutative, we call it a commutative ring. Also, rings are not required to have multiplicative inverses. If a ring does have inverses under multiplication, then we call it a division ring. This is a very descriptive name because the presence of multiplicative inverses allows us to define division. Division rings may or may not be commutative. A classic example of a non-commutative division ring is a quaternion, a quirky, four-dimensional analog to complex numbers. But if a division ring is commutative, then we call it a field.